Please wait while we process your payment
If you don't see it, please check your spam folder. Sometimes it can end up there.
If you don't see it, please check your spam folder. Sometimes it can end up there.
Please wait while we process your payment
Get instant, ad-free access to our grade-boosting study tools with a 7-day free trial!
Learn more



This site is protected by reCAPTCHA and the Google Privacy Policy and Terms of Service apply.
Create Account
Select Plan
Payment Info
Start 7-Day Free Trial!

Annual
2-49 accounts
$22.49/year + tax
50-99 accounts
$20.99/year + tax
Select Quantity
Price per seat
$29.99 $--.--
Subtotal
$-.--
Want 100 or more? Request a customized plan
You could save over 50%
by choosing an Annual Plan!

SAVE OVER 50%
compared to the monthly price!
| Focused-studying | ||
| PLUS Study Tools | ||
| AP® Test Prep PLUS | ||
| My PLUS Activity | ||
$22.49/month + tax
Save 25%
on 2-49 accounts
$20.99/month + tax
Save 30%
on 50-99 accounts
| Focused-studying | ||
| PLUS Study Tools | ||
| AP® Test Prep PLUS | ||
| My PLUS Activity | ||
No Fear provides access to Shakespeare for students who normally couldn’t (or wouldn’t) read his plays. It’s also a very useful tool when trying to explain Shakespeare’s wordplay!
Erika M.
I tutor high school students in a variety of subjects. Having access to the literature translations helps me to stay informed about the various assignments. Your summaries and translations are invaluable.
Kathy B.
Teaching Shakespeare to today's generation can be challenging. No Fear helps a ton with understanding the crux of the text.
Kay H.
No Fear provides access to Shakespeare for students who normally couldn’t (or wouldn’t) read his plays. It’s also a very useful tool when trying to explain Shakespeare’s wordplay!
Erika M.
I tutor high school students in a variety of subjects. Having access to the literature translations helps me to stay informed about the various assignments. Your summaries and translations are invaluable.
Kathy B.
Teaching Shakespeare to today's generation can be challenging. No Fear helps a ton with understanding the crux of the text.
Kay H.
Create Account
Select Plan
Payment Info
Start 7-Day Free Trial!
You will only be charged after the completion of the 7-day free trial.
If you cancel your account before the free trial is over, you will not be charged.
You will only be charged after the completion of the 7-day free trial. If you cancel your account before the free trial is over, you will not be charged.
Order Summary
Annual
7-day Free Trial
SparkNotes PLUS
$29.99 / year
Annual
Quantity
51
PLUS Group Discount
$29.99 $29.99 / seat
Tax
$0.00
SPARK25
-$1.25
25% Off
Total billed on Nov 7, 2024 after 7-day free trail
$29.99
Total billed
$0.00
Due Today
$0.00
Promo code
This is not a valid promo code
Card Details
By placing your order you agree to our terms of service and privacy policy.
By saving your payment information you allow SparkNotes to charge you for future payments in accordance with their terms.
Powered by stripe
Legal
Google pay.......



Please wait while we process your payment

Sorry, you must enter a valid email address
By entering an email, you agree to our privacy policy.
Please wait while we process your payment

Sorry, you must enter a valid email address
By entering an email, you agree to our privacy policy.
Please wait while we process your payment

Your PLUS subscription has expired
Please wait while we process your payment
Please wait while we process your payment

The Area Below a Polar Curve
Polar coordinates provide an alternate way of specifying a point in the plane. The polar coordinates [r, θ] represent the point at a distance r from the origin, rotated θ radians counterclockwise from the positive x-axis. Since r represents a distance, it is typically positive. Sometimes, r is allowed to be negative; in this case [r, θ] represents the reflection about the origin of the point [| r|, θ].
It follows from basic trigonometry that the point with polar coordinates [r, θ] has Cartesian coordinates
| (r cosθ, r sinθ) |
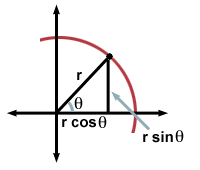
Going the other direction, the point with Cartesian coordinates (x, y) has polar coordinates
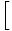 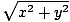 , tan-1 , tan-1 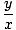   |
if it lies in quadrants I or IV and polar coordinates
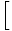 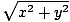 , tan-1 , tan-1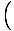 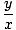 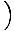 + Π + Π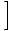 |
if it lies in quadrants II or III.
A polar function r(θ) has a graph consisting of the points [r(θ), θ]. Such a graph is known as a polar curve. One of the simplest polar curves is the circle, the graph of the polar function r(θ) = c, for some constant c. In the remainder of this section, we investigate how to find the area enclosed by a polar curve from one value of θ to another. For example, we might wish to find the area of the region below the curve r(θ) = 1 from θ = 0 to θ = Π/2 (this region is of course a quarter of the interior of a unit circle).
Considering the general case, the idea is similar to the idea for finding the area below the graph of a function in Cartesian coordinates. In that case, we approximated the region by a bunch of thin rectangles; here, we approximate it by thin circular sectors (shaped like slices of pie).
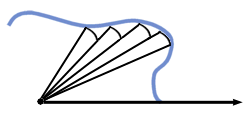
Such a method worked before because we knew beforehand how to compute the area of a rectangle. Now we attempt this computation for a circular sector. Suppose the sector has angular width of Δθ and is part of a circle of radius r, with area Πr2. Since the sector accounts for Δθ/2Π of the area of the circle, the area of the sector is equal to
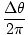 Πr2 = Πr2 = 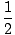 (Δθ)r2 (Δθ)r2 |
Summing together the areas of all the thin sectors and taking the limit as Δθ→ 0 (and the number of sectors approaches infinity), we get the definite integral
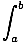 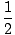 r(θ)2dθ r(θ)2dθ |
Note that, because of the square in the expression being integrated, the integral counts all area as positive, even when r(θ) < 0.
Applying this theory to the example given above, we get an area of
 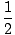 (1)2dθ = (1)2dθ =  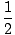 θ θ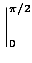 = = 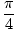 |
which is indeed one quarter of the area of a unit circle.
Please wait while we process your payment





