Chemical Potential  -  The Chemical Potential is defined such that:

.

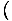
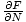
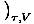Enthalpy  -  The Enthalpy, H, is defined as H = U = pv and its identity is dH = τ dσ + V dp + μ dN.

Extensive  -  An extensive variable of a system doubles upon duplication of the system. Important extensive variables are the volume V, the entropy σ, the number N and the energy U. Compare to intensive variables.

Gibbs Free Energy  -  The Gibbs Free Energy, G, is defined as G = U - τσ + pV and its identity is dG = - σ dτ + V dp + μ dN.

Helmholtz Free Energy  -  The Helmholtz Free Energy, F, is defined as F = U - τσ and its identity is dF = - σ dτ - p dV + μ dN.

Intensive  -  An intensive variable of a system remains constant upon duplication of the system. Important intensive variables are the temperature τ, the chemical potential μ, and the pressure p. Compare to extensive variables.

Legendre Transform  -  The Legendre Transform is a mathematical tool that we employ to change variables in expression of then energy, such as defining F = U - τσ in order to change variables from σ to τ in the energy.

Maxwell Relations  -  The Maxwell Relations give relationships between the partial derivative of one variable with respect to a variable in a different pairing and the corresponding cross partial derivative of the other variable in the second pairing with respect to the other variable in the first.

Pressure  -  The pressure is defined such that: p = -

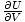
, and is one of the important intensive variables in thermodynamics.

Thermodynamic Identity  -  The Thermodynamic Identity relates the energy U to the 6 variables we have discussed: