Please wait while we process your payment
If you don't see it, please check your spam folder. Sometimes it can end up there.
If you don't see it, please check your spam folder. Sometimes it can end up there.
Please wait while we process your payment
Get instant, ad-free access to our grade-boosting study tools with a 7-day free trial!
Learn more



This site is protected by reCAPTCHA and the Google Privacy Policy and Terms of Service apply.
Create Account
Select Plan
Payment Info
Start 7-Day Free Trial!

Annual
2-49 accounts
$22.49/year + tax
50-99 accounts
$20.99/year + tax
Select Quantity
Price per seat
$29.99 $--.--
Subtotal
$-.--
Want 100 or more? Request a customized plan
You could save over 50%
by choosing an Annual Plan!

SAVE OVER 50%
compared to the monthly price!
| Focused-studying | ||
| PLUS Study Tools | ||
| AP® Test Prep PLUS | ||
| My PLUS Activity | ||
$22.49/month + tax
Save 25%
on 2-49 accounts
$20.99/month + tax
Save 30%
on 50-99 accounts
| Focused-studying | ||
| PLUS Study Tools | ||
| AP® Test Prep PLUS | ||
| My PLUS Activity | ||
No Fear provides access to Shakespeare for students who normally couldn’t (or wouldn’t) read his plays. It’s also a very useful tool when trying to explain Shakespeare’s wordplay!
Erika M.
I tutor high school students in a variety of subjects. Having access to the literature translations helps me to stay informed about the various assignments. Your summaries and translations are invaluable.
Kathy B.
Teaching Shakespeare to today's generation can be challenging. No Fear helps a ton with understanding the crux of the text.
Kay H.
No Fear provides access to Shakespeare for students who normally couldn’t (or wouldn’t) read his plays. It’s also a very useful tool when trying to explain Shakespeare’s wordplay!
Erika M.
I tutor high school students in a variety of subjects. Having access to the literature translations helps me to stay informed about the various assignments. Your summaries and translations are invaluable.
Kathy B.
Teaching Shakespeare to today's generation can be challenging. No Fear helps a ton with understanding the crux of the text.
Kay H.
Create Account
Select Plan
Payment Info
Start 7-Day Free Trial!
You will only be charged after the completion of the 7-day free trial.
If you cancel your account before the free trial is over, you will not be charged.
You will only be charged after the completion of the 7-day free trial. If you cancel your account before the free trial is over, you will not be charged.
Order Summary
Annual
7-day Free Trial
SparkNotes PLUS
$29.99 / year
Annual
Quantity
51
PLUS Group Discount
$29.99 $29.99 / seat
Tax
$0.00
SPARK25
-$1.25
25% Off
Total billed on Nov 7, 2024 after 7-day free trail
$29.99
Total billed
$0.00
Due Today
$0.00
Promo code
This is not a valid promo code
Card Details
By placing your order you agree to our terms of service and privacy policy.
By saving your payment information you allow SparkNotes to charge you for future payments in accordance with their terms.
Powered by stripe
Legal
Google pay.......



Please wait while we process your payment

Sorry, you must enter a valid email address
By entering an email, you agree to our privacy policy.
Please wait while we process your payment

Sorry, you must enter a valid email address
By entering an email, you agree to our privacy policy.
Please wait while we process your payment

Your PLUS subscription has expired
Please wait while we process your payment
Please wait while we process your payment

The pH of Non-Buffered Solutions
When a strong acid or a strong base is added to water, it nearly completely dissociates into its ion constituents because it has a pKa or pKb less than zero. For example, a solution of H2SO4 in water contains mostly H+ and SO42-, and almost no H2SO4 is left undissolved. This makes calculating the pH of a strong acid or strong base solution exceedingly simple--the concentration of acid equals the concentration of H+. Recall that pH is computed by taking the negative log of of [H+]. Common strong acids that should be memorized include HCl (hydrochloric), HNO3 (nitric), HClO4 (perchloric), and H2SO4 (sulfuric). Strong bases include Group I hydroxides (LiOH, NaOH, KOH, etc.) and Group II hydroxides except for Be(OH)2 and Ba(OH)2.
Calculating the pH of weak acid and weak base solutions is much more complicated than the above case--weak acids and bases do not completely dissociate in aqueous solution but are in equilibrium with their dissociated forms. Therefore, we must apply what we know about equilibria to solve these types of problems. For example, let's calculate the pH of a 0.10 M solution of acetic acid in water. To do this, we first write down the equilibrium involved and the expression for the equilibrium constant:

Next, you should compile a table of values for the concentration of all species involved in the equilibrium. We already know that the initial concentration, [ ]o, of acetic acid is 0.10 M and that the initial concentration of H+ is 10-7 (since the solvent is neutral water). Even though there is an initial concentration of H+ in solution, it is so small compared to the amount produced by the acid that it is usually ignored. From the stoichiometry of the reaction, one mole of H+ and one mole of acetate (Ac-) are produced for each mole of acetic acid dissociated. Therefore, if we denote the amount of acetic acid dissociated as x, the final concentrations of H+ and Ac- are both x, and the final concentration of HAc in solution is 0.10 M - x. This data is summarized in :
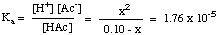
After you have compiled the table of values, you can substitute the equilibrium concentration values for each species into your expression for Ka as shown below:

You should note that the above equation is a quadratic equation in x and therefore requires use of the quadratic equation to solve for x. If, however, we can make the assumption that [HAc]o - x = [HAc]o, the equation becomes much easier to solve. We can do this if HAc is a weak enough acid that it dissociates very little, and the change in [HAc] is negligible.
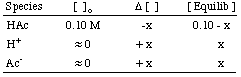
Is this assumption valid? Solving the quadratic equation using the quadratic formula gives a pH of 2.88. The difference of 0.01 pH unit is small enough to be insignificant, so the assumption is valid in this case and will certainly save you some time on a test. We can make the approximation [HA]o - x = [HA]o so long as x is less than 5% of the initial concentration of HA. X will be greater than 5% of [HA]o with stronger weak acids at low concentrations. Consider these guidelines when you decide whether or not to make the approximation: you can simplify the quadratic equation if the solution is more concentrated than 0.01 M and the pKa of the acid is greater than 3.
Please wait while we process your payment





