Continuity and Limits
Terms
Continuity
-
A function is continuous at a point if the three following conditions are met:
1)
f (a)
is defined. 2)
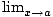f (x)
exists. 3)
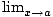f (x) = f (a)
. A conceptual way to describe continuity is this: A function is
continuous if its graph can be traced with a pen without lifting the pen from
the page.
f (x)
exists. 3)
f (x) = f (a)
. A conceptual way to describe continuity is this: A function is
continuous if its graph can be traced with a pen without lifting the pen from
the page.
Infinite Discontinuity
-
A category of discontinuity in which a vertical
asymptote exists at
x = a
and
f (a)
is
undefined.
Jump Discontinuity
-
A category of discontinuity in which
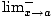f (x)≠
f (x)
, but both of these limits exist and are finite.
f (x)≠
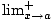f (x)
, but both of these limits exist and are finite.
Limit
-
The value
A
to which a function
f (x)
gets arbitrarily close as the value of
the independent variable
x
gets arbitrarily close to a given value
a
. Such
a limit is symbolized this way:
lim
xâÜ’a
f (x) = A
.
One-Sided Limit
-
A limit based entirely on the values of a function taken at an
x
-value
slightly greater than or less than a given value. Whereas a two-sided limit
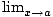f (x)
takes into account the values of
x
near
a
which are
both greater than and less than
a
, a one-sided limit from the left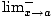
f (x)
or from the right
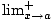f (x)
takes into account only
values of
x
less than
a
, or greater than
a
, respectively.
f (x)
takes into account the values of
x
near
a
which are
both greater than and less than
a
, a one-sided limit from the left
f (x)
or from the right
f (x)
takes into account only
values of
x
less than
a
, or greater than
a
, respectively.
Point Discontinuity
-
A category of discontinuity in which a function has a well-defined two-sided
limit at
x = a
, but either
f (x)
is not defined at
a
or its value at
a
is not equal to this limit.
