Thermodynamics: Energy, Concentration, and Potential

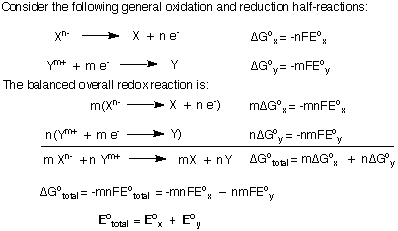
Figure %: Proof of the Additivity of Oxidation and Reduction Potentials

Note that in the that the ΔG's were added together and then we solved for E^o_total instead of simply adding the E^o's. As that proof shows, if there are no "left-over" electr ons in the overall balanced equation, then you can sum the potentials of the half-reactions.

However, if you are trying to add two reduction potentials to generate the reduction potential of a new reaction, your balanced equation will have some left-over electrons and you cannot simply add the two reduction potentials together. I will derive the formula for adding reduction or oxidation potentials together to generate a new half-reaction. I will use the reduction of Fe³⁺ to Fe metal as my example. Be sure to understand the important conclusion that when summing reduction potentials, E^o_total does not equal the sum of the individual E^o's.

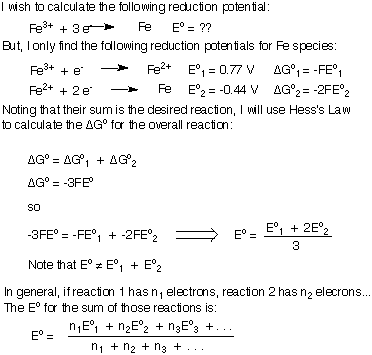
Figure %: Derivation of the Rule for Adding Reduction Potentials

The Nernst Equation--Concentration Effects

So far in our discussion of electrochemical cells, we have only considered reactions at "standard state" which are, in reality, impossible to achieve. The moment you hook up the wire connecting two half-cells the reaction proceeds and changes the concentrations of all reactants and products. Furthermore, if the reaction is exothermic or endothermic, the reaction mixture will heat or cool making it deviate from the standard temperature. Therefore, we need a way to relate E^o at the standard conditions and E, the potential at any real condition. That relationship, called the Nernst Equation, was first derived by Walther Nernst and earned him the 1920 Nobel Prize in chemistry. The is found below:

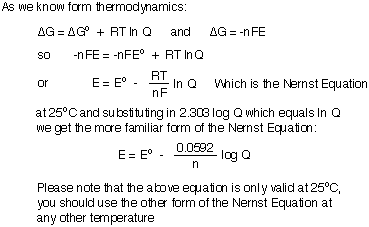
Figure %: Derivation of the Nernst Equation

Please note that the familiar form of the Nernst Equation is only applicable when the reaction is carried out at 25^oC (298^oK). At any other temperature you need to use the first form of the Nernst Equation: E = E^o - (RT/nF) ln Q. One caveat in using the Nernst Equation: Q is the reaction quotient, so you must have already balanced the redox reaction to be able to place the correct power on each concentration term in Q. Make sure you use consistent units for both R and T!

As you can tell by inspection of the Nernst equation the cell potential depends on concentration. In fact, the equation implies directly that you can construct a galvanic cell with half-cells of identical composition but differing concentrations--a concentration cell. As is intuitively obvious from our knowledge of osmotic pressure a concentration cell reacts in such a manner as to dilute the more concentrated half-cell and to concentrate the more dilute half-cell as shown in .

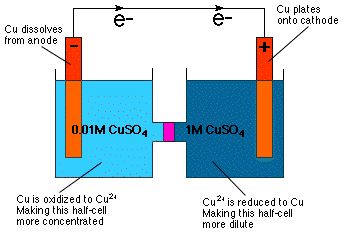
Figure %: Depiction of a Concentration Cell

As shown in the , the dilution of the cathode half-cell is achieved by reducing Cu²⁺ to Cu metal and plating that metal onto the Cu electrode. In the anode half-cell, the Cu anode is oxidized to Cu²⁺ and, thus, dissolved into the solution, making the anode cell more concentrated.