payment page
Problem :
Four billiard balls, each of mass .5 kg, all are traveling in the same direction on a billiard table, with speeds 2 m/s, 4 m/s, 8 m/s and 10 m/s. What is the linear momentum of this system?
The linear momentum of a system is simply the sum of the linear momentum of the constituent parts. Thus we only need to find the momentum of each ball:
Problem :
A 60 kg man standing on a stationary 40 kg boat throws a .2 kg baseball with a velocity of 50 m/s. With what speed does the boat move after the man throws the ball? Assume no friction between the man and the boat.
We begin by designating our system as the man, the ball and the boat. Initially all are at rest, so the linear momentum of the system is zero. When the man throws the ball, no external force is acting upon the system, so linear momentum must be conserved. Thus the man and boat must move in a direction opposite to the direction of travel of the ball. When thrown, the ball is given a linear momentum of p = mv = 10. Thus the man and boat, with a total mass of 100 kg, must also have a linear momentum of 10, but in the opposite direction. Since we are trying to find v, we can state that v = p/m = 10/100 = .1 m/s. The man and boat move with this small velocity of .1 m/s.
Problem :
A .05 kg bullet is fired at a velocity of 500 m/s, and embeds itself in a block of mass 4 kg, initially at rest and on a frictionless surface. What is the final velocity of the block?
Again, we use the principle of conservation of momentum. The bullet is the only object with initial velocity, to the initial momentum of the bullet-block system is: p = mv = 25. Once the bullet embeds itself in the block, the block and bullet must have the same momentum of 25. Thus: v = p/m = 25/4.05 = 6.17 m/s. Note that the mass used in the calculation was 4.02 kg, as the bullet became embedded in the block, and added to its total mass.
Problem :
An object at rest explodes into three pieces. Two, each of the same mass, fly off in different directions with velocity 50 m/s and 100 m/s, respectively. A third piece is also formed in the explosion, and has twice the mass of the first two pieces. What is the magnitude and direction of its velocity?
The object is initially at rest, and no forces act on the system during the explosion, so the total linear momentum of zero must be conserved. Firstly, we denote the positive direction as the direction that the piece going 100 m/s travels. Thus if we sum the linear momentum of the first two pieces, we find: P12 = 100m - 50m = 50m. The third piece, with a mass of 2m, must provide momentum in the opposite direction to ensure that the total momentum of the system is zero:
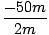= - 25
Problem :
A spaceship moving at 1000 m/s fires a missile of mass 1000 kg at a speed of 10000 m/s. What is the mass of the spaceship it slows down to a velocity of 910 m/s?
Recall that momentum, like energy, is relative and depends on the velocity of the observer. For simplicity's sake, let us use the reference frame of the spaceship. Thus, in this frame, the spaceship is initially at rest, fires the missile at a speed of 10000 - 1000 = 9000 m/s, and then moves backwards at a speed of 90 m/s. Initially in this frame, the total momentum of the system is zero. The missile, when fired, is given a momentum of (1000 kg)(9000 m/s) = 9×106. Thus the spaceship must move backwards with the same momentum, if momentum is to be conserved. Thus we know the final speed of the spaceship, and the final momentum, and we can calculate the mass:
= 
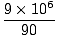= 1×105 kg
