A complete Lewis structure should include both bonding and formal charge information. Therefore, the structure of NO2- should be drawn as shown in .

Resonance Structures
While drawing the , you may have noticed that the two oxygens appear to be different. One bears a negative charge and has only one bond to N while the other is neutral and doubly bonded to N. Why should these two oxygens be different? There is absolutely no reason why they should be. It is impossible to make a single Lewis structure that depicts the equivalency of the two oxygens. Instead, we can represent NO2- as a hybrid of two resonance structures as shown in .
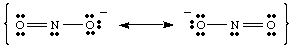
It is important to note that NO2- is neither one nor the other resonance structure but is the average of the two. A good analogy for resonance structures is found in mixing colors. Green is neither yellow nor blue but is a mixture of the two colors, just as NO2- is neither of the resonance forms but is a mixture of the two structures.
When more than one reasonable Lewis structure can be drawn for a molecule, the actual structure of the molecule will be a resonance hybrid of the structures.
Exceptions to the Octet Rule
Although the octet rule has allowed us to draw almost every conceivable Lewis structure, there are certain molecules that do not obey the octet rule. In this section, we will point out the most common exceptions.
Boron and aluminum compounds commonly place only six electrons around the metal center. For example, AlH3 has only six electrons on Al. Compounds with less than an octet (or duet for H) of electrons around each atom are called electron deficient. Boron and aluminum compounds are frequently electron deficient while compounds involving most other elements are not. The reason why boron and aluminum can form electron deficient compounds has to do with their low electronegativities. Because both atoms are not very electronegative, they are not terribly unhappy when they have fewer electrons than they require for full octets.
While boron and aluminum can have less than a full octet, some atoms like phosphorous and atoms in period three or below on the periodic table (greater period numbers) can exceed their octets. Try to draw a reasonable Lewis structure for either PF5 or SF6. You should not find it possible to obey the octet rule on either phosphorous or sulfur. Frequently textbooks say that atoms like P and S are able to expand their octets by letting the extra electrons fill their empty 3d orbitals. Your chemistry course may even require you to memorize this "fact". However, this description of the bonding in such compounds is completely false. After you have read Molecular Orbital Theory you should be able to come up with a better reason. The explanation of the expanded octet must wait until then due to is complexity. For now, realize that atoms below period two may expand their octets to accommodate more than eight electrons.
Valence Shell Electron Pair Repulsion Theory
When drawing Lewis structures, only bonding and charge information is available. Such structures tell us absolutely nothing about the real three-dimensional shapes that molecules have. To determine molecular geometry, chemists use Valence Shell Electron Pair Repulsion theory-- abbreviated VSEPR. The VSEPR model makes the reasonable assumption that electron pairs repel each other. Therefore, electron pairs in bonds and lone pairs will want to be oriented as far away from each other as possible. By analyzing all possible combinations of lone pairs and bonding pairs we can predict the structure of any covalent molecule. , , , and show the results of such an analysis. (The tables are broken down into four parts due to the sizes of the images and not because there are any fundamental differences between the tables.) For each, A stands for the central atom, B stands for any other atoms bonded to A, and e stands for the lone pairs on the central atom.

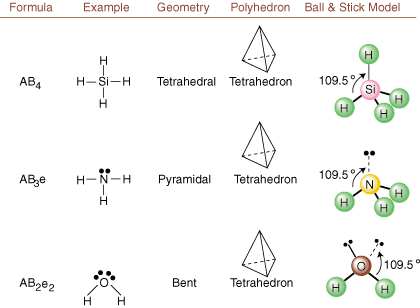

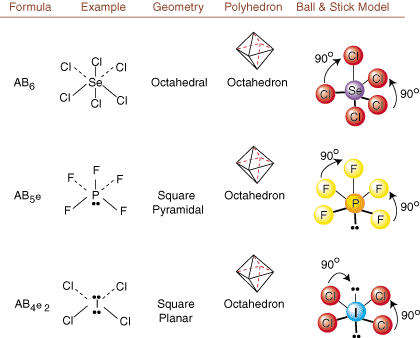
By comparing a Lewis structure to the examples provided in the above figures, you can predict the geometries of many molecules accurately. Note in the tables that the lone pair groups e are placed at positions to minimize interactions with other groups e or B; the lone pairs take up these positions preferentially when you must choose to place either e or B there. For example, for the molecule AB3e2 in , the e's are placed in the equatorial positions where they are at 90 and 120 degree angles from other groups, rather than at the axial positions where they would be restricted to 90 degree angle interactions. We can understand this trend by visualizing the condensed electron density of a lone pair near an atomic center, in comparison with a bonded electron pair in which electron density is distributed between two atoms.
VSEPR theory does not work well for transition metals. To predict their geometries you will need a more advanced treatment of bonding which will not be presented in this SparkNote.
To predict the geometries of polycentric molecules (those with A greater than one), simply use the above tables of geometries to predict the geometry of each center independently of others. For example, to predict the geometry of HOCH2NH2, you need only predict the geometry at oxygen, carbon, and nitrogen. To do so, first draw the Lewis structure as shown in :
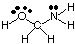
Next, classify the VSEPR type for each atom bonded to more than one other atom using A, B, and e. Oxygen is AB2e2, so it is bent. Carbon is AB4, so it is tetrahedral. Nitrogen is AB3e, so it is pyramidal. Now you can draw the structure of HOCH2NH2 in three dimensions.


 payment page
payment page



