Molecular Orbitals: Molecular Orbital Theory

Previous Next

To demonstrate why it is important to take the number of antibonding electrons into account in our bond order calculation, let us consider the possibility of making a molecule of He₂. An orbital correlation diagram for He₂ is provided in :

Figure %: An orbital correlation diagram for a hypothetical He-He molecule

From the orbital correlation diagram above you should notice that the amount of stabilization due to bonding is equal to the amount of destabilization due to antibonding, because there are two electrons in the bonding orbital and two electrons in the antibonding orbital. Therefore, there is no net stabilization due to bonding so the He₂ molecule will not exist. The bond order calculation shows that there will be a bond order of zero for the He₂ molecule--exactly what we should predict given that helium is a noble gas and does not form covalent compounds.

Both hydrogen and helium only have 1s atomic orbitals so they produce very simple correlation diagrams. However, we have already developed the techniques necessary to draw a correlation diagram for a more complex homonuclear diatomic like diboron, B₂. Before we can draw a correlation diagram for B₂, we must first find the in-phase and out-of-phase overlap combinations for boron's atomic orbitals. Then, we rank them in order of increasing energy. Each boron atom has one 2s and three 2p valence orbitals. Due to the great difference in energy between the 2s and 2p orbitals, we can ignore the overlap of these orbitals with each other. All orbitals composed primarily of the 2s orbitals will be lower in energy than those comprised of the 2p orbitals. shows the process of creating the molecular orbitals for diboron by combining orbitals of atomic boron. Note that the orbitals of lowest energy have the most constructive overlap (fewest nodes) and the orbitals with the highest energy have the most destructive overlap (most nodes).

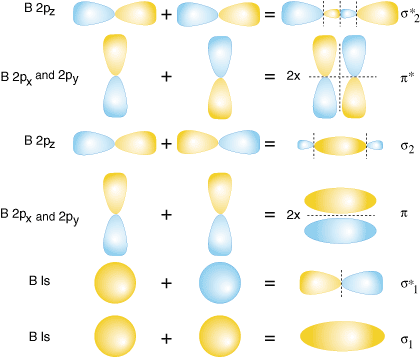
Figure %: The molecular orbitals of diboron

Notice that there are two different kinds of overlap for p-orbitals--end-on and side-on types of overlap. For the p-orbitals, there is one end-on overlap possible which occurs between the two p_z. Two side-on overlaps are possible--one between the two p_x and one between the two p _y. P-orbitals overlapping end-on create s bonds. When p-orbitals bond in a side-on fashion, they create p bonds. The difference between a p bond and a s bond is the symmetry of the molecular orbital produced. s bonds are cylindrically symmetric about the bonding axis, the z-direction. That means one can rotate the s bond about the z-axis and the bond remains the same. In contrast, p bonds lack that cylindrical symmetry and have a node passing through the bonding axis.

Now that we have determined the energy levels for B₂, let's draw the orbital correlation diagram ():

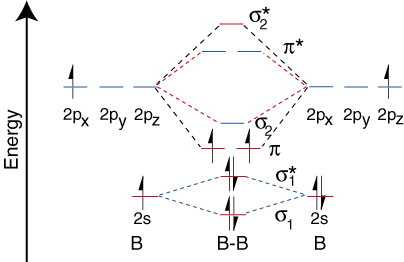
Figure %: Orbital correlation diagram for diboron

The orbital correlation diagram for diboron, however, is not generally applicable for all homonuclear diatomic molecules. It turns out that only when the bond lengths are relatively short (as in B₂, C₂, and N₂) can the two p-orbitals on the bonded atoms efficiently overlap to form a strong p bond. Some textbooks explain this observation in terms of a concept called s-p mixing. For any atom with an atomic number greater than seven, the p bond is less stable and higher in energy than is the s bond formed by the two end-on overlapping p orbitals. Therefore, the following orbital correlation diagram for fluorine is representative of all homonuclear diatomic molecules with atomic numbers greater than seven.