Reaction Kinetics: Reaction Mechanisms: Mechanisms of Chemical Reactions

Properties of Mechanisms

Mechanisms describe in a stepwise manner the exact collisions and events that are required for the conversion of reactants into products. Mechanisms achieve that goal by breaking up the overall balanced chemical equation into a series of elementary steps. An elementary step is written to mean a single collision or molecular vibration that results in a chemical reaction. The following picture of an elementary step shows a single collision between water and boron trifluoride:

Figure %: Schematic representation of an elementary step

The molecularity of an elementary step describes the number of reactive partners in the elementary step. For example, the above elementary step is called bimolecular because two molecules collide. Commonly, elementary steps are mono-, bi-, or termolecular. The probability of four molecules colliding at exactly the same place and time is so small that we can safely assume that no reaction will ever be tetramolecular. Because take up a large amount of space, we will represent elementary steps in this SparkNote as normal reactions with molecular formula line equations. You will know from the context (i.e. talking about the steps of a mechanism) whether the reaction is an elementary step or an overall reaction.

To better understand mechanisms, let's consider the following mechanism for the decomposition of ozone, O₃:

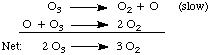
The above mechanism exhibits a property of all mechanisms: it is a series of elementary steps whose sum is the overall balanced reaction. Note the presence of the oxygen atom, O, intermediate in the above equation. It is an intermediate because it is both created and destroyed in the mechanism and does not appear in the net equation.

Another property of mechanisms is that they must predict the experimentally determined rate law. To calculate the rate law from a mechanism you need to first know the rate limiting step. The rate limiting step determines the rate of the reaction because it is the slowest step. You can rationalize that a reaction can only go so fast as its slowest step by thinking about what happens when you encounter an accident on the highway that closes all but one lane. You may have been able to race along at 65 m.p.h. (depending on your state's laws) before you reached the lane closure but the slow passage of cars past the accident limits your rate. You can only go as fast through that one lane as the slowest car in front of you.

In the above , the first reaction is labeled as "slow". This reaction is the rate determining step because it is the slowest step. As we have stated, that means that the rate of the overall reaction is equal to the rate of the rate determining step. The rate of an elementary step is the rate constant for that step multiplied by the concentrations of the reactants raised to their stoichiometric powers. Note that this rule only applies for elementary steps. The rate of an overall reaction is NOT the product of the concentrations of the reactants raised to their stoichiometric powers. The rate law for the first elementary step in the is rate = k [O₃]. Because this step is the rate determining step, the rate law is also the rate law for the overall reaction. Using similar techniques we can calculate the rate law predicted by any mechanism. We then check the predicted rate law against the experimentally determined rate law to test the validity of the proposed mechanism.