Previous Next
Figure %: Initial rates data for the bromination of acetone

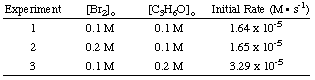
To calculate the order of the reaction for bromine, notice that experiments 1 and 2 hold the concentration of acetone constant while doubling the concentration of bromine. The initial rate of the reaction is unaffected by the increase in bromine concentration, so the reaction is zero order in bromine. We can prove this mathematically by taking the ratio of the rates from experiments 1 and two:

As you can see in the above equations, by holding the concentrations of all but one species constant between two experiments, you can calculate the order of the reaction in a single reactant at a time. By similar reasoning, we can conclude that because the rate of reaction doubled when the concentration of acetone was doubled (cf. experiments 1 and 3) the reaction must be first order in acetone. However, had the rate quadrupled or octupled with a doubling of the acetone concentration, the reaction would have been second or third order in acetone, respectively. In practice, you will likely never see a reaction with an order higher than 3. If you calculate an order higher than 3 for a reaction, double check your math because that is highly unusual. If you compute a fractional power for a reactant's order, do not be discouraged; they are quite common (especially half-order reactions).

To calculate the value of k, the rate constant, you simply plug into the rate law the values of the concentrations, the orders, and the rate of the reaction from any one of the three experiments. All three experiments should give a value of 1.64 x 10-4 s-1. You should prove this to yourself.

Using the Integrated Rate Law to Determine the Rate Law

One major disadvantage to using the method of initial rates is the need to perform multiple experiments. Another disadvantage is that it works only for relatively slow reactions. If your reaction proceeds too fast, the rate that you measure will have a large range of uncertainty. To combat these problems, chemists have developed a method that uses data on concentration versus time during a reaction to infer the order of a reaction. The strategy works by comparing concentration versus time information to the mathematical predictions made by integrated rate laws. This is usually accomplished by assuming that a reaction has a certain order and making a plot out of the data that should be linear if the assumption about the rate law is correct. If we guess correctly, the graph is linear. If we are wrong, then the graph is curved and we need to choose another order against which to plot our data.

To practice this method, we will first need to know the forms of the integrated rate laws for some common reaction orders. Luckily, only orders zero through two are common so we need to only consider reactions with these three orders. Due to the mathematical complexities of the problem, we will only consider rate laws of the form rate = [A]n. However, with some experimental tricks I will explain below, that treatment does allow us to use the method of integrated rates for a reaction with any arbitrary rate law.

By integrating the differential rate law (the simple rate law from Heading ), we derive the integrated rate law for a reaction. Without proof, we present below the forms of the integrated rate laws for reaction orders zero through two:

Previous page Determining the Rate Law page 1 Next page Determining the Rate Law page 3

Take a Study Break