Did you know you can highlight text to take a note? x

Any discussion of energy must be prefaced with one of the fundamental statements of physics: energy is always conserved. This guiding principle forms the basis for many branches of physics. That said, though total energy in a system cannot change in total amount, energy can change forms. Electrical energy can turn into mechanical energy; mechanical energy can turn into heat. However, since at this point we are only familiar with mechanical energy, for now we can only use the principle of the conservation of energy if no energy is converted to other forms. That is, for our purposes, all mechanical energy must remain mechanical energy. In order to know when mechanical energy is conserved, we must define those forces that do conserve mechanical energy.

Definition of a Conservative Force

So exactly what kinds of forces conserve mechanical energy? To answer this we consider particles traveling in closed loops under the influence of the forces in question. In other words, a closed loop describes a "round trip", during which the particle is under influence of the force. Many systems produce closed loops, such as a ball bouncing up and down, or a mass on a spring. If a conservative force acts upon the particle during this closed loop, the velocity of the particle at the beginning and the end of the loop must be the same. Why? Because if the velocity is any different, the kinetic energy of the particle will be different, meaning that mechanical energy must not have been conserved. Thus we come to our first statement about conservative forces:

If a body is under the action of a force that does no net work during any closed loop, then the force is conservative. If work is done, the force is nonconservative.

In other words, a particle located at the same physical location in a closed loop must have the same kinetic energy at all times if it is within a conservative system. This fact is the fundamental definition of a conservative force. Though we will derive other properties of conservative forces from this statement, it remains the most important one to keep in mind.

Since the work over a closed loop must be zero for conservative forces, what other properties can we state? Let's break the path of a closed loop into two separate paths:

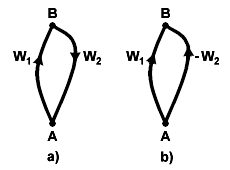
Figure %: a) A closed loop, split into 2 segments. b) Two different paths from point A to point B.
Since the path in part a) is a closed loop, we know that the total work around the loop must be zero if the force in question is conservative: W1 + W2 = 0. Now compare the two different paths taken from A to B in part b). The work on the first path is the same as part a), simply W1. The direction of travel on the second path is reversed in b), implying that the work done over the path is negated, or equal to - W2. But we know from a) that - W2 = W1. Thus the work done over path 1 and path 2 in part b) is the same! This concept, called path independence, is incredibly useful, as we will soon see. Stated verbally:
The work done by a conservative force in moving a body from an initial location to a final location is independent of the path taken between the two points

Let's examine the implications of this statement. Consider a particle moving between two points in an odd shaped path. Our old definition of work demands that we evaluate the work done at each part of the odd path in order to evaluate the total work done over the journey, and thus the change in kinetic energy and velocity. With this just-stated principle of conservative forces, however, we can use any path we like: a straight line, a circular arc, or a path in which the work done on the particle is constant. Though our first statement about conservative forces is powerful, this second statement proves to be the most applicable: we will use this concept to solve numerous problems in the sections to come.

Examples of Conservative and Nonconservative Forces

Such abstract principles might be confusing. In order to clarify these two very important concepts, we will examine two forces: gravity, a conservative force, and friction, a nonconservative one.

Previous section Next page Conservative vs. Nonconservative Forces page 2

Take a Study Break